Mindlin solution on ground deformation caused by the trench excavation during installation of concrete diaphragm wall panels

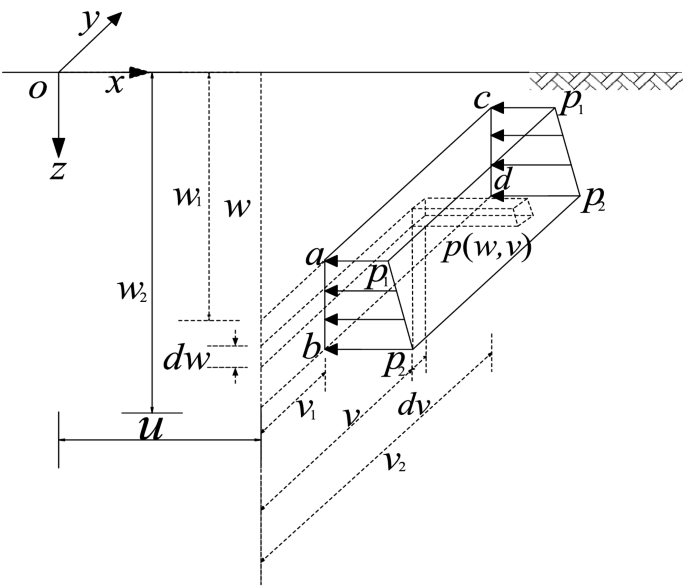
Making use of Mindlin answer²⁰, inside an elastic infinite half area, the horizontal displacement δ_x and vertical displacement δ_z¹⁸ at any location N(x, y, z) beneath the motion of a horizontal concentrated load P at any location M(u, v, w) might be expressed in Eqs. (1) and (2), The schematic view of location N and M is proven in Fig. 1.

$$ delta_{{textual content{x}}} = frac{P}{beta }left{ {left( {3 – 4nu } proper)left( {frac{1}{{R_{1} }} + frac{{X^{2} }}{{R_{2}^{3} }}} proper) + frac{1}{{R_{2} }} + frac{{X^{2} }}{{R_{1}^{3} }} + frac{2wz}{{R_{2}^{3} }}left( {1 – frac{{3X^{2} }}{{R_{2}^{2} }}} proper)} proper.left. { + frac{{4left( {1 – nu } proper)left( {1 – 2nu } proper)}}{{R_{2} + Z_{2} }}left[ {1 – frac{{X^{2} }}{{R_{2} (R_{2} + Z_{2} )}}} right]} proper} $$

(1)

$$ delta_{z} = frac{PX}{beta }left[ {frac{{left( {3 – 4nu } right)Z_{1} }}{{R_{2}^{3} }} + frac{{Z_{1} }}{{R_{1}^{3} }} – frac{{6wzZ_{2} }}{{R_{2}^{5} }} + frac{{4left( {1 – nu } right)left( {1 – 2nu } right)}}{{R_{2} (R_{2} + Z_{2} )}}} right] $$

(2)

the place: E is Younger’s modulus, (nu) is Poisson’s ratio, (beta = 8pi Efrac{1 – nu }{{1 + nu }}), (R_{i} = sqrt {X^{2} + Y^{2} + Z_{i}^{2} } (i, = ,1 , or , 2),X = x – u,Y = y – v,Z_{2} = z + w,Z_{1} = z – w,)

Horizontal displacement

Assuming the slurry and groundwater on the identical degree on the floor floor, the slurry strain follows a triangular distribution from the bottom floor alongside depth. Inside a sure vary of depth beneath the bottom floor, the slurry strain follows a trapezoidal distribution on the ditch wall to stability the lateral hydrostatic and soil strain. The unit drive strain on the facet of the diaphragm wall is proven in Eq. (3).

$$ P = frac{1}{{(w_{2} – w_{1} )}}left[ {(w_{2} p_{1} – w_{1} p_{2} ) + (p_{2} – p_{1} )w} right]dvdw $$

(3)

The parameters within the Eq. (3) are offered in Fig. 2. w₁, w₂ are the higher and decrease limits of the slurry depth vary, v is the wide selection, p_{i(i=1, 2)} is the distinction between slurry strain and lateral static earth strain, as proven in Eq. (4).

$$ p_{i} = K_{0} (gamma_{sat} – gamma_{w} )z + (gamma_{w} – gamma_{s} )z,(i, = ,1,2) $$

(4)

the place, γ _s is unit weight of slurry, γ_sat is the saturated unit weight of soil, γ _w is the unit weightof water, and Ok₀ is the coefficient of at-rest earth strain.

As proven in Fig. 2, the Eqs. (1) and (3) are built-in on the bounded closed area U(v₁ ≤ v ≤ v₂, w₁ ≤ w ≤ w₂), the outcomes are proven in Eq. (5).

$$ delta_{x} = frac{{left( {p_{2} – p_{1} } proper)}}{{beta left( {w_{2} – w_{1} } proper)}}left[ {left{ {G1_{x} (w,v)} right}} right.left. {_{{w = w_{1} }}^{{w = w_{2} }} } right]_{{v = v_{1} }}^{{v = v_{2} }} + frac{{left( {p_{1} w_{2} – p_{2} w_{1} } proper)}}{{beta left( {w_{2} – w_{1} } proper)}}left[ {left{ {G2_{x} (w,v)} right}} right.left. {_{{w = w_{1} }}^{{w = w_{2} }} } right]_{{v = v_{1} }}^{{v = v_{2} }} $$

(5)

the place:

$$ start{aligned} G1_{x} (w,v) & = left( {3 – 4nu } proper)left{ {log left( {R_{1} + Y} proper)left( {frac{1}{2}left( {X^{2} + Z_{1}^{2} } proper) + zZ_{1} } proper)} proper. + Yzlog left( {R_{1} + Z_{1} } proper) + frac{{R_{1} Y}}{2} hfill & quad + left. {Xleft( {wT_{x2} + zT_{x1} + zT_{xy} } proper) – vz} proper} + frac{1}{2}left( {Z_{1} Z_{2} – X^{2} } proper)log left( {R_{2} + Y} proper) – Yzlog left( {R_{2} + Z_{2} } proper) hfill & quad + frac{{R_{2} Y}}{2} – Xzleft( {T_{xy} – T_{x2} } proper) – XZ_{2} T_{x2} + Yz + left{ {zR_{2} left( {frac{{w^{2} X^{2} Y}}{{R_{2}^{2} Z_{2} left( {X^{2} + Z_{2}^{2} } proper)}} – } proper.} proper.left. {frac{{Yz^{2} }}{{Z_{2} left( {X^{2} + Y^{2} } proper)}}} proper) hfill & quad+ z(Ylog left( {R_{2} + Z_{2} } proper) + left( {Z_{1} + Z_{2} } proper)log left( {R_{2} + Y} proper) + left. {XT_{xy} – v – 2XT_{x2} )} proper} hfill & quad – frac{{2left( {1 – nu } proper)left( {1 – 2nu } proper)}}{3}left{ {left( {3left( {Yz + YR_{2} } proper. + } proper.} proper.left. {left. {frac{{left( {2w – z} proper)Z_{2}^{2} left( {T_{x2} – Z_{2} } proper)}}{X}} proper) – 3zZ_{2} log left[ {Y + R_{2} } right] – 3Y} proper) hfill & quad instances zlog left[ {Z_{2} + R_{2} } right] + left( {3wZ_{2} + X^{2} } proper)log left[ {Y + R_{2} } right] + frac{{Yleft( {2w – z} proper)left( {w + z} proper)left( {R_{2} – w – z} proper)}}{{left( {X^{2} + Y^{2} } proper)}} hfill & quad – frac{{left( {2w – z} proper)left( {w + z} proper)^{2} T_{xy} }}{X} – left. {frac{{left( { – 2w^{3} – 3w^{2} z + 3X^{2} z + z^{3} } proper)T_{x2} }}{X} – frac{{2log left[ {Y + R_{2} } right]}}{{X^{2} }}} proper} , hfill finish{aligned} $$

(6)

$$ start{aligned} G2_{x} (w,v) & = left( {1 – 2nu } proper)left( {frac{{R_{2} XT_{2X} }}{{R_{2} }} + Ylog left( {R_{1} + Z_{1} } proper) + Z_{1} } proper. instances log left( {R_{1} + Y} proper) – w + XT_{{xz_{1} }} + left. {frac{{X^{2} YT_{1x} }}{XY}} proper) hfill & quad + Ylog left( {R_{2} + Z_{2} } proper) + Z_{2} log left( {R_{2} + Y} proper) + XT_{xy} + XT_{1x} – XT_{2x} – v hfill & quad+ 2z instances left( {frac{{R_{2} Yz}}{{Z_{2} left( {X^{2} + Y^{2} } proper)}} – log left( {R_{2} + Y} proper) + frac{{wXT_{2x} }}{{Z_{2}^{2} }}} proper) + 2left( {1 – nu } proper)left( {1 – 2nu } proper) hfill & quad instances left( {frac{{left( {Z_{2}^{2} – X^{2} } proper)left( {T_{x2} – T_{xy} } proper)}}{X}} proper. – v + 2Z_{2} log left[ {Y + R_{2} } right]Ylog left[ {Z_{2} + R_{2} } right] hfill & quad left. { – frac{{Yleft( {R_{2} – Z_{2} } proper)Z_{2} }}{{X^{2} + Y^{2} }} + frac{{Z_{2}^{2} T_{xy} }}{X} – frac{{left( {Z_{2}^{2} + X^{2} } proper)T_{x2} }}{X}} proper) hfill finish{aligned} $$

(7)

the place: (T_{alpha i} = arctan frac{{XYZ_{i} }}{{alpha^{2} R_{i} }}) (α = X, Y or Z_i; i = 1 or 2) ; (T_{AB} = arctan frac{B}{A}).

In keeping with the idea of double particular integral,

$$ left[ {left{ {Gi_{m} (w,v)} right}} right.left. {_{{w = w_{1} }}^{{w = w_{2} }} } right]_{{v = v_{1} }}^{{v = v_{2} }} = Gi_{m} (w_{1} ,v_{1} ) + Gi_{m} (w_{2} ,v_{2} ) – Gi_{m} (w_{1} ,v_{2} ) – Gi_{m} (w_{2} ,v_{1} ),(i, = ,1,2;m, = ,x,y) $$

(8)

Eqs. (5) to (7) might be solved in line with Eq. (8), and the horizontal displacement beneath the horizontal trapezoidal distributed load in semi-infinite area might be obtained.

Vertical displacement

$$ delta_{z} = frac{{left( {p_{2} – p_{1} } proper)X}}{{beta left( {w_{2} – w_{1} } proper)}}left[ {left{ {G1_{z} (w,v)} right}} right.left. {_{{w = w_{1} }}^{{w = w_{2} }} } right]_{{v = v_{1} }}^{{v = v_{2} }} + frac{{Xleft( {p_{1} w_{2} – p_{2} w_{1} } proper)}}{{beta left( {w_{2} – w_{1} } proper)}}left[ {left{ {G2_{z} (w,v)} right}} right.left. {_{{w = w_{1} }}^{{w = w_{2} }} } right]_{{v = v_{1} }}^{{v = v_{2} }} $$

(9)

the place:

$$ start{aligned} G1_{z} (w,v) & = left( {4nu – 3} proper)left( {left( {3zln left( {R_{2} + Y} proper) + Yln left( {R_{2} + Z_{2} } proper) – v} proper) – } proper.left. {frac{{T_{x2} left( {X^{2} – 2z^{2} } proper)}}{x} + XT_{xy} } proper) hfill & quad- { 2}zleft( { – frac{{w^{2} Y}}{{R_{2} left( {X^{2} + Z_{2}^{2} } proper)}} – 2ln left( {R_{2} + Y} proper)} proper.left. { – frac{{2zT_{x2} }}{X}} proper) + 2left( {nu – 1} proper)left( {2nu – 1} proper)left( {2zln left( {R_{2} + Y} proper)} proper. hfill & quad left. { + frac{{left( {Ylog left( {R_{2} + Z_{2} } proper) – v} proper) + left( {X^{2} – Z_{1} Z_{2} } proper)left( {T_{xy} – T_{x2} } proper)}}{X}} proper) hfill & quad + left( {zln left( {R_{1} + Y} proper) + Yln left( {R_{1} + Z_{1} } proper) + XT_{x1} – XT_{xy} + v} proper) hfill finish{aligned} $$

(10)

$$ start{aligned} G2_{z} (w,v) & = 2zleft( {frac{wY}{{R_{2} left( {X^{2} + Z_{2}^{2} } proper)}} – frac{{T_{x2} }}{X}} proper) + 4left( {nu – 1} proper)left( {2nu – 1} proper)left( {frac{{Z_{2} left( {T_{xy} – T_{x2} } proper)}}{X} – ln left( {R_{2} + Y} proper)} proper) hfill & quad + left( {left( {3 – 4nu } proper)left( {ln left( {R_{2} + Y} proper) + frac{{2zT_{x2} }}{X}} proper) + ln left( {R_{1} + Y} proper)} proper) hfill finish{aligned} $$

(11)

The parameter definition within the equation is similar as that in Eq. (7). In keeping with Eq. (8), the vertical displacement of Eq. (9) to (11) beneath horizontal trapezoid distributed load in semi-infinite area might be achieved.

Validation

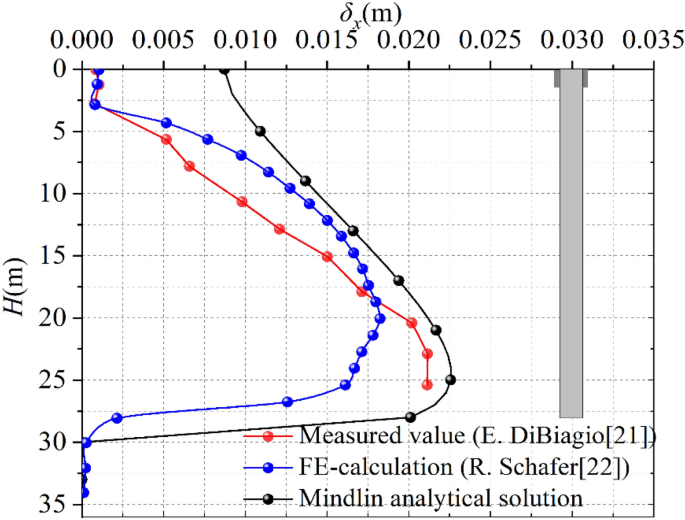
With a view to confirm the validity of the Mindlin answer, this paper takes the complete scale discipline check of a slurry-trench excavation in Oslo, Norway because the background²¹, which particularly investigated the deformations of the encompassing floor and the soundness of the ditch throughout the trench excavation and a subsequent resting interval beneath slurry help, There have been 14 gauges positioned to find out soil actions, 10 alongside the vertical centerline of the ditch and 5 in a horizontal aircraft at a depth of 15.5 m. R. Schafer generated a three-dimensional finite-element mannequin for the simulation of the slurry-trench excavation in Oslo²². On this paper Mindlin answer is in contrast with the measurements of the slurry trench check excavation in Oslo²¹ and the calculated soil actions²². Horizontal displacement comparability alongside the vertical centerline of the ditch is proven in Fig. 3, and horizontal actions at a depth of 15.5 m is proven in Fig. 4.

The check panel reveals a depth of D = 28 m, a size of L = 5 m and a width of W = 1 m and was excavated utilizing a slurry with an unit weight of 10.8 kN/m³. Parameter “H” is excavation depth of the ditch. The check panel was in a clay-filled canyon which is typical of the Oslo area, most of soil layer parameters on the web site are decided by totally different in situ and laboratory testings. Ok₀, coefficient of at-rest earth strain, was measured by the hydraulic fracturing methodology at totally different elevations. The weighted common values of the parameters of every soil layer are thought of as γ = 18.8 kN/m³, E = 20 MPa, v = 0.35, Ok₀ = 0.65, a extra detailed description is given by R. Schafer²².

The Finite-element mannequin generated by R. Schafer consisted of about 7500 trilinear brick parts incorporating a coupled calculation of the pore water circulate and the efficient stress discipline.

It may be seen from Fig. 3, Mindlin analytical answer is of vertical spoon form. Due to the ditch excavation, the horizontal deformation of the soil increasd with the depth of the ditch. The measured most displacement happens at 25.4 m, the displacement is 21.1 mm, and the utmost displacement of Mindlin answer happens at 24 m, about 5/6 D (D is the depth of the groove), the utmost displacement is 22.57 mm, the place which the utmost vertical displacement occured is comparable, and the utmost displacement error is 6.5%. It may get that the Mindlin analytical answer agrees properly with the FE- calculation by R. Schafer²² and the change development of the sector measured worth²¹.

Determine 4 illustrates the horizontal displacement comparability of the ditch facet at a depth of 15.5 m. It may be seen from Fig. 4, the horizontal displacement (at a depth of 15.5 m) presents a U-shaped curve with massive displacement within the center and small displacement on either side alongside the lengthy facet of the ditch. The utmost displacement happens at y = 0. The measured most displacement is 15.23 mm, the utmost displacement of Mindlin answer is 16.26 mm, and the utmost displacement of finite component answer is 16.09 mm. The positions of those three most displacements are related, and the utmost displacement error is 6.3%. It may be noticed that the Mindlin analytical answer is in good settlement with the measured worth and FE- calculation.

The above evaluation reveals that it’s possible to make use of Mindlin answer to resolve the formation deformation brought on by the ditch formation of concrete diaphragm wall.

Source link